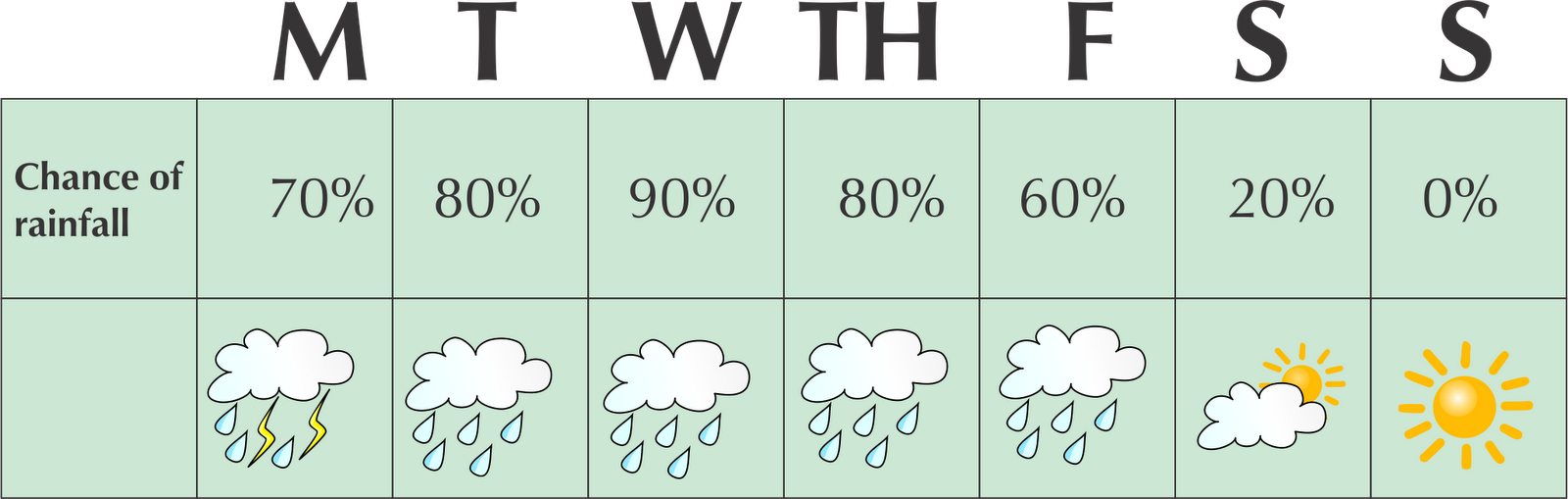
When one enters some games reserves and national parks in South Africa managed by SA National Parks you will find a sign in the form of a pie chart indicating the risk of a fire occurring in the park that particular day. Look at the picture of one of these fire risk pie charts and determine what weather predictions the fire risk probability is based upon.

The fire risk probability depends on different aspects of the weather - primarily the probability of rain and high wind speeds. For example, if it has been very dry, and it is predicted that it will be windy, the risk of fire increases. If there is a \(\text{90}\%\) chance of rain predicted, the risk if fire is reduced.