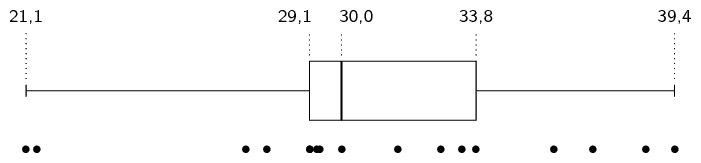
\(\text{30}\) ; \(\text{21,4}\) ; \(\text{39,4}\) ; \(\text{33,4}\) ; \(\text{21,1}\) ; \(\text{29,3}\) ; \(\text{32,8}\) ; \(\text{31,6}\) ; \(\text{36}\) ;
\(\text{27,9}\) ; \(\text{27,3}\) ; \(\text{29,4}\) ; \(\text{29,1}\) ; \(\text{38,6}\) ; \(\text{33,8}\) ; \(\text{29,1}\) ; \(\text{37,1}\)
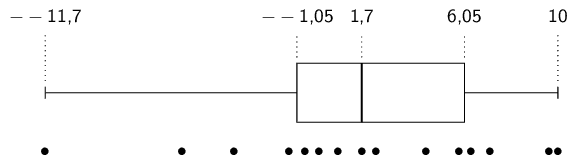
Below is the box-and-whisker diagram of the data as well as dots representing the data themselves. Note that learners do not neeed to draw the dots, but this helps us to see that there are two outliers on the left.